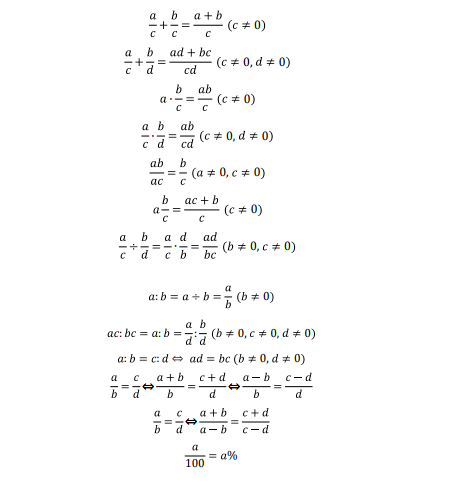AMC8是由美国数学协会(MAA)主办的一项面向初中生的国际性数学竞赛。它不仅为学生提供了一个展示自己数学才能的平台,也为未来参加更高级别的数学竞赛如 AMC10 和 AMC12 奠定了基础。
一、AMC8 适合的学生群体
1.已经就读国际学校的学生
目的:通过挑战基础的 AMC8 考试,为接下来的 AMC10/12 打下坚实的基础。
优势:熟悉国际竞赛模式,提前适应更高难度的数学问题,增强自信心。
2.将来计划就读国际学校的学生
目的:希望通过 AMC8 成绩证明自己的数学能力,增加申请国际学校的竞争力。
优势:一份优秀的 AMC8 成绩可以作为个人学术能力的重要佐证,帮助在众多申请者中脱颖而出。
3.计划就读英美高中的学生
目的:使用 AMC8 作为国际初中数学的衔接,针对性练习,也可以提前适应高中数学课程模式。
优势:有助于过渡到更具挑战性的高中数学课程,同时培养解决问题的能力。
4.想要提升数学能力的学生
目的:激发学生对数学的兴趣,无论未来的升学规划如何,都可以将 AMC8 的经历作为潜在的优势。
优势:无论是体制内还是国际教育体系,AMC8 都能有效提升数学思维能力和解题技巧。
二、AMC8 报名与考试信息
报名时间
截止日期:2026年1月
注意事项:想要参加 AMC8 的同学请尽快完成报名,避免错过机会。
考试时间
日期:2026年1月
时间安排:具体考试时间段需根据主办方通知为准,请关注官方公告。
参赛资格
年级要求:8年级或以下
年龄限制:不超过14.5岁
竞赛时长
总时长:40分钟
竞赛题型
题量:25道单项选择题
内容范围:涵盖代数、几何、数论、组合等基础数学知识领域
三、备考建议
1.基础知识巩固
重点复习:确保对代数、几何、数论、组合四大模块的基本概念和公式有扎实的理解。
教材推荐:《Art of Problem Solving》系列书籍是很好的学习资源,尤其是 Volume 1。
2.真题练习
历年真题:通过做历年的 AMC8 真题来熟悉题型和考试节奏,了解常见的出题思路。
模拟测试:定期进行限时模拟测试,提高答题速度和准确性。
3.解题技巧训练
快速解题法:学习并掌握一些常用的快速解题技巧,如排除法、特殊值法等。
错题分析:建立错题本,记录每次练习中的错误,并进行详细分析,找出错误原因并加以改进。
4.心理准备
保持冷静:考试过程中遇到难题不要慌张,合理分配时间,优先保证会做的题目得分。
适度放松:考前一周调整作息,保证充足的睡眠,以最佳状态迎接考试。
不知道孩子数学水平如何?
扫码进行AMC8能力测评,看看孩子是否适合参加AMC8!