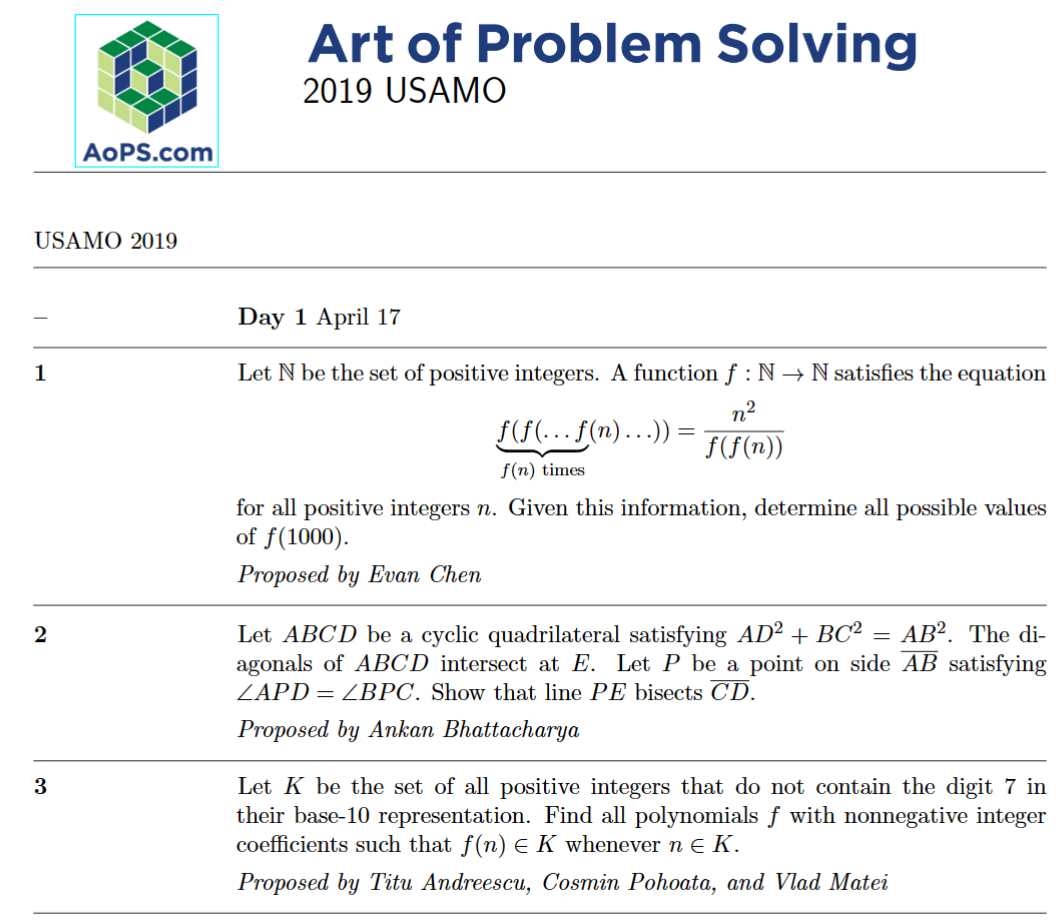
2019年USAMO 真题及答案

2019年USAMO 真题：

Day 1

Note: For any geometry problem whose statement begins with an asterisk $(*)$, the first page of the solution must be a large, in-scale, clearly labeled diagram. Failure to meet this requirement will result in an automatic 1-point deduction.

Problem 1

Let $\mathbb{N}$ be the set of positive integers. A function $f:\mathbb{N}\to\mathbb{N}$ satisfies the equation\[\underbrace{f(f(\ldots f}_{f(n)\text{ times}}(n)\ldots))=\frac{n^2}{f(f(n))}\]for all positive integers $n$. Given this information, determine all possible values of $f(1000)$.

Problem 2

Let $ABCD$ be a cyclic quadrilateral satisfying $AD^2 + BC^2 = AB^2$. The diagonals of $ABCD$ intersect at $E$. Let $P$ be a point on side $\overline{AB}$ satisfying $\angle APD = \angle BPC$. Show that line $PE$ bisects $\overline{CD}$.

Problem 3

Let $K$ be the set of all positive integers that do not contain the digit $7$ in their base-$10$ representation. Find all polynomials $f$ with nonnegative integer coefficients such that $f(n)\in K$ whenever $n\in K$.

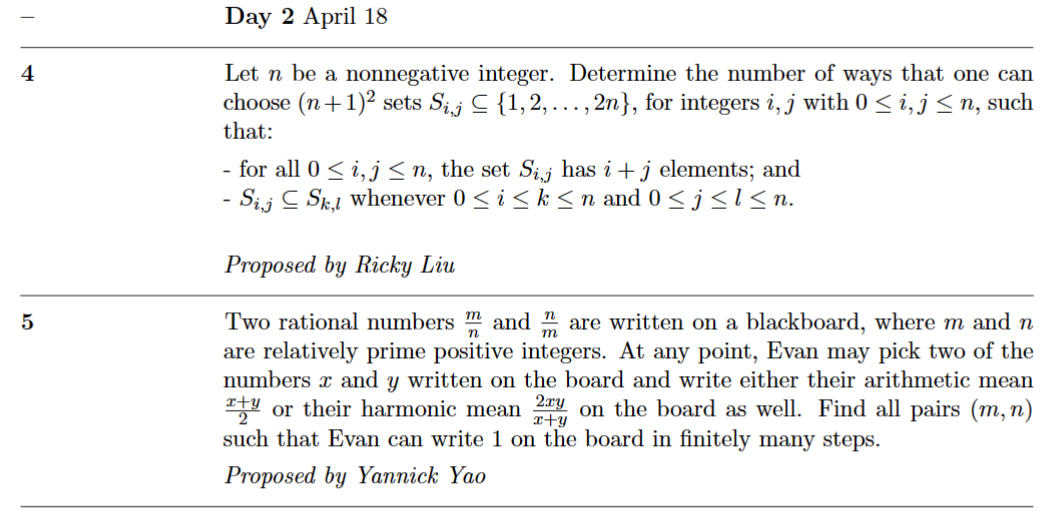
Day 2

Problem 4

Let $n$ be a nonnegative integer. Determine the number of ways that one can choose $(n+1)^2$ sets $S_{i,j}\subseteq\{1,2,\ldots,2n\}$, for integers $i,j$ with $0\leq i,j\leq n$, such that: for all $0\leq i,j\leq n$, the set $S_{i,j}$ has $i+j$ elements; and $S_{i,j}\subseteq S_{k,l}$ whenever $0\leq i\leq k\leq n$ and $0\leq j\leq l\leq n$.

以下是我们为您整理的真题试卷，扫码即可免费领取完整版：

更多USAMO 历年真题+真题详解
扫码添加顾问即可免费领取