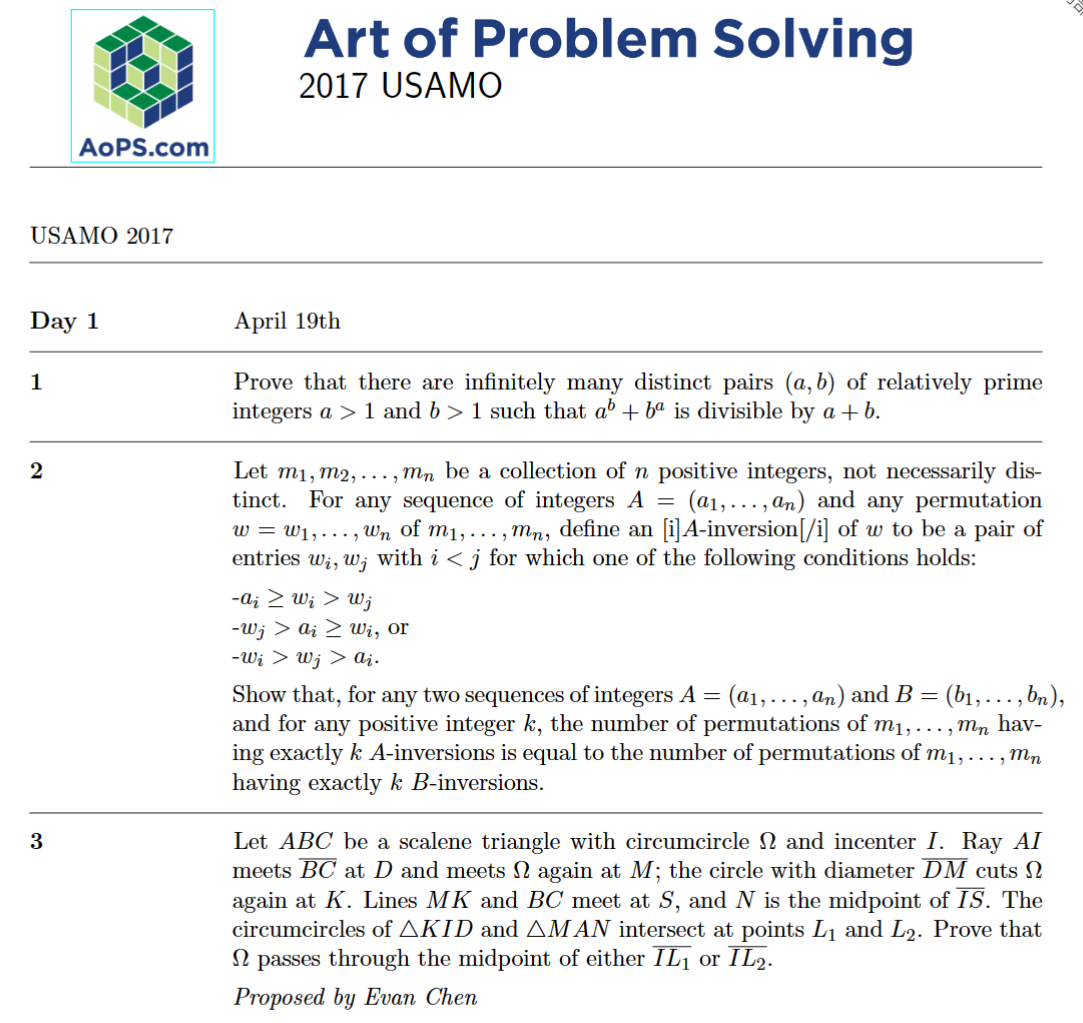
2017年USAMO 真题及答案

2017年USAMO 真题：

Day 1

Note: For any geometry problem whose statement begins with an asterisk ($*$), the first page of the solution must be a large, in-scale, clearly labeled diagram. Failure to meet this requirement will result in an automatic 1-point deduction.

Problem 1

Prove that there are infinitely many distinct pairs $(a,b)$ of relatively prime positive integers $a > 1$ and $b > 1$ such that $a^b + b^a$ is divisible by $a + b.$

Problem 2

Let $m_1, m_2, \ldots, m_n$ be a collection of $n$ positive integers, not necessarily distinct. For any sequence of integers $A = (a_1, \ldots, a_n)$ and any permutation $w = w_1, \ldots, w_n$ of $m_1, \ldots, m_n$, define an $A$-inversion of $w$ to be a pair of entries $w_i, w_j$ with $i < j$ for which one of the following conditions holds:\[a_i \ge w_i > w_j,\]\[w_j > a_i \ge w_i,\]or\[w_i > w_j > a_i.\]Show that, for any two sequences of integers $A = (a_1, \ldots, a_n)$ and $B = (b_1, \ldots, b_n)$, and for any positive integer $k$, the number of permutations of $m_1, \ldots, m_n$ having exactly $k$ $A$-inversions is equal to the number of permutations of $m_1, \ldots, m_n$ having exactly $k$ $B$-inversions.

Problem 3

($*$) Let $ABC$ be a scalene triangle with circumcircle $\Omega$ and incenter $I$. Ray $AI$ meets $\overline{BC}$ at $D$ and meets $\Omega$ again at $M$; the circle with diameter $\overline{DM}$ cuts $\Omega$ again at $K$. Lines $MK$ and $BC$ meet at $S$, and $N$ is the midpoint of $\overline{IS}$. The circumcircles of $\triangle KID$ and $\triangle MAN$ intersect at points $L_1$ and $L_2$. Prove that $\Omega$ passes through the midpoint of either $\overline{IL_1}$ or $\overline{IL_2}$.

Day 2

Note: For any geometry problem whose statement begins with an asterisk ($*$), the first page of the solution must be a large, in-scale, clearly labeled diagram. Failure to meet this requirement will result in an automatic 1-point deduction.

Problem 4

Let $P_1$, $P_2$, $\dots$, $P_{2n}$ be $2n$ distinct points on the unit circle $x^2+y^2=1$, other than $(1,0)$. Each point is colored either red or blue, with exactly $n$ red points and $n$ blue points. Let $R_1$, $R_2$, $\dots$, $R_n$ be any ordering of the red points. Let $B_1$ be the nearest blue point to $R_1$ traveling counterclockwise around the circle starting from $R_1$. Then let $B_2$ be the nearest of the remaining blue points to $R_2$ travelling counterclockwise around the circle from $R_2$, and so on, until we have labeled all of the blue points $B_1, \dots, B_n$. Show that the number of counterclockwise arcs of the form $R_i \to B_i$ that contain the point $(1,0)$ is independent of the way we chose the ordering $R_1, \dots, R_n$ of the red points.

以下是我们为您整理的真题试卷，扫码即可免费领取完整版：

更多USAMO 历年真题+真题详解
扫码添加顾问即可免费领取